函數 (Function)

令 $(X, d_x)$ , $(Y, d_y)$ 為兩個 metric spaces

函數的定義

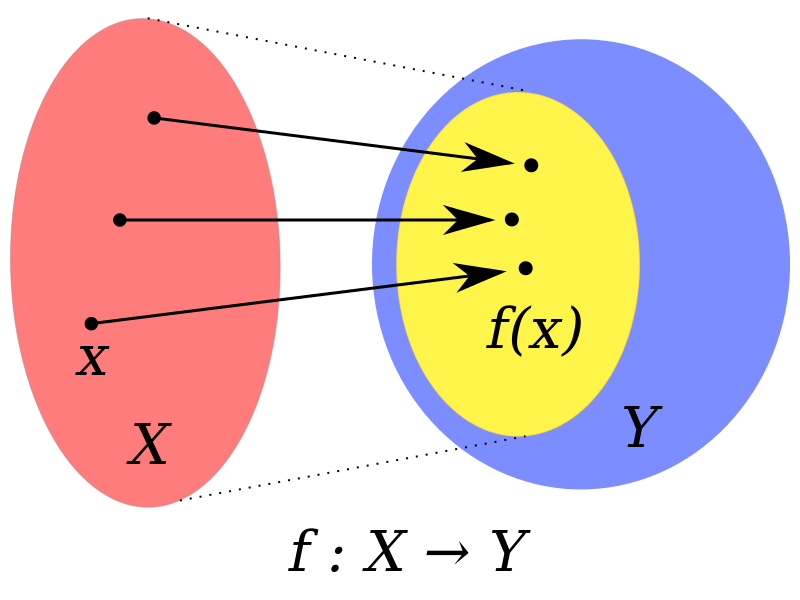
$f$ is a function if $\forall x \in X$ $\exists \; !y \in Y$ $\ni$ $f(x)=y$ .
$X$ 為 $f$ 的定義域(domain)，記為 $X=\text{dom} f$ .
$f(X)$ 為 $f$ 的值域(range)， $f(X) = \{ y\in Y \; \vert \; y=f(x), \forall x \in X\}$ .
依上述定義， $f$ 可為一對一或是多對一函數，但不可為一對多函數。
- (one-to-one, bijection) $\forall x_1, x_2 \in X$ $\ni$ $f(x_1)=y_1$ and $f(x_2)=y_2$ then $y_2=y_1$ $\rightarrow$ $x_1 = x_2$ .
- (onto, surjection) $\forall y \in Y$ $\exists x \in X$ $\ni$ $f(x)=y$ .
  - $\because f(X) \subseteq Y$ , 而onto為 $f(X)=Y$ ，此時函數可能為one-to-one或many-to-one.
  - $\exists x\in X$ $\ni$ $f(x)=y_1$ and $f(x)=y_2$ but $y_1 \neq y_2$ 不符合函數定義。
一對多的情形在代數中稱為映射(mapping)或是關係(relation)。
函數必須是one-to-one時，inverse relation $f^{-1}$ 才是函數。因one-to-one時才可避免invese時one-to-many的情形發生。
若集合 $X$ 與集合 $Y$ 間存在1-1的函數時，稱兩集合有相同的基數(cardinal number)，即兩集合的元素個數相同；或稱兩集合等價(equivalent)，此時該1-1函數為等價關係(equivalence relation)，即滿足reflexive, symmetric, transitive。
E.g. 自然數 $\mathbb{N}$ 與整數 $\mathbb{Z}$ 為等價集合。
E.g. $\forall a, b \in \mathbb{R}$ ， $(a,b)$ 與 $(0,1$ 為等價集合。
E.g. $(0,1)$ 與實數 $\mathbb{R}$ 為等價集合。

If $E \subset X$ , $f(E) = \lbrace f(x) \vert \ x \in E \rbrace$ , the $f(E)$ is the image of $E$ .
If $F \subset Y$ , $f^{-1}(F) = \lbrace x \in X \vert f(x) \in F \rbrace$ , $f^{-1}$ is the pre-image (inverse image) of $F$
E.g. $f:\lbrace 1,2,3 \rbrace \rightarrow \lbrace a,b,c,d \rbrace$ .
- $f(x) \equiv \lbrace \begin{array}{ll} a & x=1 \\ a & x=2 \\ c & x=3 \end{array}$
- The image $f( \lbrace 2,3 \rbrace ) = \lbrace a,c \rbrace$ .
E.g. $f: \mathbb{R} \rightarrow \mathbb{R}$ , and $f(x) = x^2$ .

$\begin{array}{rcl} f^{-1}([4,9]) & = & \lbrace x \in \mathbb{R} \vert f(x) \in [4,9] \rbrace \\ & = & \lbrace x \in \mathbb{R} \vert 4 \leq x^2 \leq 9 \rbrace \\ & = & \lbrace x \in \mathbb{R} \vert 2 \leq x \leq 3 \text{ or } -3 \leq x \leq -2 \rbrace \\ & = & [-3, -2] \cup [2,3]. \end{array}$
- 若令 $f: \mathbb{R}^{+} \rightarrow \mathbb{R}$ , 則 $f^{-1}([4,9]) = [2,3]$ .
Theorem: Every infinite subuset of a countable set $S$ is countable.
由定理可知，一個可數的集合之子集合必為可數集合，而不可為不可數集合。

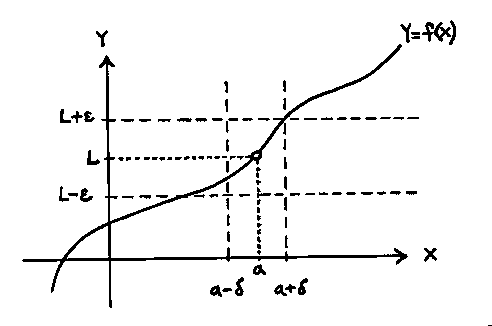
$f: X \rightarrow Y$ is a function, $f$ is continuous at a point $x \in X$ if
- $\forall \epsilon > 0$ $\exists \ \delta > 0$ $\ni$ $d_Y(f(x_1), f(x_2)) < \delta$ $\; \forall d_X(x_1, x_2) < \epsilon$ .
如果 $f$ 在集合 $S$ 中每一點都連續時，稱 $f$ is continous on $S$ 。
下圖為 $X=Y=\mathbb{R}$ 的範例。

- 函數 $f: X \times Y \rightarrow \mathbb{R}$ 有上界。
- 則 $\sup_{x,y} f(x,y) = \sup_x \sup_y f(x,y) = \sup_y \sup_x f(x,y)$ .

- 函數 $f: X \times Y \rightarrow \mathbb{R}$ 之值域為有界集合。
- 則 $\sup_y \inf_x f(x,y) \leq \inf_x \sup_y f(x,y)$ .

Let $E \subseteq X$ , and a function $f: E \rightarrow Y$ .
$p$ is a limit point of $E$ , and it is written $\lim_{x \rightarrow p} f(x)=q$ if
- $\forall \epsilon > 0$ $\exists \delta > 0$ $\ni$ $d_Y(f(x), q) < \epsilon$ $\forall x \in E$ $d_X(x,p) < \delta$ .
注意， $p \in X$ ，但 $p$ 不一定在集合 $E$ 中。(如 $\sqrt{2}$ 不在有理數集合中)。
For every sequence ${p_n} \in E$ such that $p_n \neq p$ and $\lim_{n \rightarrow \infty} p_n = p$ , then $\lim_{x \rightarrow p} f(x) = q$ $\Leftrightarrow$ $\lim_{n \rightarrow \infty} f(p_n) = q$ .
If $f$ has two limit points at $p_1$ and $p_2$ , then $p_1=p_2$ . (極限點唯一存在)
Theorem: $f: X \rightarrow Y$ is continuous function if $f^{-1}(U)$ is open set for every open set $U \subset Y$ .